Gravitation Class 11 Important Extra Questions Physics Chapter 8

Gravitation Class 11 Important Extra Questions Physics Chapter 8

IMP QUE 11TH PHY
Published on By Amresh Academy

   

Gravitation Important Extra Questions Very Short Answer Type

Question 1.
What velocity will you give to a donkey and what velocity to a monkey so that both escape the gravitational field of Earth?
Answer:
We will give them the same velocity as escape velocity is independent of the mass of the body.

Question 2.
How does Earth retain most of the atmosphere?
Answer:
Due to force of gravity.

Question 3.
Earth is continuously pulling the moon towards its center. Why does not then, the moon falls on the Earth?
Answer:
The gravitational force between the Earth and the moon provides the necessary centripetal force to the moon to move around the Earth. This centripetal force avoids the moon to fall onto the Earth.

Question 4.
Which is greater out of the following:
(a) The attraction of Earth for 5 kg of copper.
(b) The attraction of 5 kg copper for Earth?
Answer:
Same.

Question 5.
Where does a body weigh more – at the surface of Earth or in a mine?
Answer:
At the surface of Earth, a body weighs more.

Question 6.
How is it that we learn more about the shape of Earth by studying the motion of an artificial satellite than by studying the motion of the moon?
Answer:
This is because an artificial satellite is closer to the Earth than Moon.

Question 7.
If the Earth is regarded as a hollow sphere, then what is the weight of an object below the surface of Earth?
Answer:
Zero.

Question 8.
What is the formula for escape velocity in terms of g and R?
Answer:
Ve = 2gR−−−−√ .

Question 9.
What is the orbital period of revolution of an artificial satellite revolving in a geostationary orbit?
Answer:
It is 24 hours.

Question 10.
Can we determine the mass of a satellite by measuring its time period?
Answer:
Yes.

Question 11.
Is it possible to put a satellite into an orbit by firing it from a huge canon?
Answer:
Tins can be possible only if we can ignore air friction and technical difficulties.

Question 12.
What is the amount of work done in bringing a mass from the surface of Earth on one side to a point diametrically opposite on the other side? Why?
Answer:
The work done is zero. Because the gravitational potential difference is zero.

Question 13.
Name one factor on which the period of revolution of a planet around the Sun depends.
Answer:
Mean distance of the planet from the Sun.

Question 14.
The gravitational potential energy of a body of mass m is -107 J. What is the energy required to project the body out of the gravitational field of Earth?
Answer:
107 J.

Question 15.
Does the force of friction and other contact forces arise due to gravitational attraction? If not, what is the origin of these forces?
Answer:
No. The contact forces have an electrical origin.

Question 16.
Two satellites are at different heights. Which would have greater orbital velocity? Why?
Answer:
The satellite at the smaller height would have greater velocity.
This is because v0 ∝ 1r√ .

Question 17.
How much energy is required by a satellite to keep it orbiting? Neglect air resistance? Why?
Answer:
No energy is required by a satellite to keep it orbiting. This is because the work done by the centripetal force is zero.

Question 18.
At noon the attractions of the Earth and Sun on a body on the surface of Earth are in opposite directions. But at midnight, they are in the same direction. Does a body weigh more at mid-night?
Answer:
No. The weight of the body is due to the Earth’s gravity only. What is the full form of the geostationary satellite APPLE? The full form of APPLE is the Ariane Passenger PayLoad Experiment.

Question 19.
What is geodesic?
Answer:
It is the shortest distance between any two points.

Question 20.
Why is G called a universal constant?
Answer:
G is called a universal constant because its value is the same everywhere.

Question 22.
Where does a body weigh more at the pole or at the equator?
Answer:
It weighs more at the pole.

Question 23.
What is the relation between orbital and escape velocity?
Answer:
ve = 2–√v0.

Question 24.
The weight of a body is 20N, What is the gravitational pull of the body on the Earth?
Answer:
20N.

Question 25.
Why Newton’s law of gravitation is said to be universal- law?
Answer:
It is called so because this law holds good irrespective of the nature of the interacting bodies at all places and at all times.

Question 26.
A particle is to be placed, in turn, outside four objects, each of mass m;
(a) a large uniform solid sphere,
(b) a large uniform spherical shell,
(c) a small uniform solid sphere,
(d) a small uniform shell.
In each situation, the distance between the particle and the center of the object is d. Rank the objects according to the magnitude of the gravitational force they apply on the particle, greatest first.
Answer:
They all apply the same gravitational force and hence all will tie.

Question 27.
Can we determine the mass of a satellite by measuring its time-period?
Answer:
No.

Question 28.
Does the gravitational force between two particles depends upon the medium between them?
Answer:
No, it does not depend upon the medium between the two particles.

Question 29.
Two artificial satellites of different masses are moving in the same orbit around the Earth. Can they have the same speed?
Answer:
Yes, they can have the same speed as orbital speed is independent of the mass of the satellite.

Question 30.
In an imaginary system, the central star has the same mass as our Sun but is much brighter so that only a planet twice the distance between Earth and the Sun can support life. Assuming biological evolution (including aging processes etc.) on that planet similar to ours, what would be the average life span of a ‘human’ on that planet in terms of its natural year? The average life span of a human on the Earth may be taken to be 70 years.
Answer:
25 planet years.

Question 31.
If the force of gravity acts on all bodies in proportion to their masses, then why does not a heavy body fall faster than a light body?
Answer:
Acceleration due to gravity is independent of the mass of the body.

Question 32.
What is the weight of a body in a geostationary satellite?
Answer:
The weight of a body is zero in a geostationary satellite.

Question 33.
A satellite does not need any fuel to circle around the Earth. Why?
Answer:
The gravitational force between satellite and Earth provides the centripetal force required by the satellite to move in a circular orbit.

Question 34.
What will be the effect on the weight of the bodies if Earth stops rotating about its axis?
Answer:
The weight of the bodies will increase.

Question 35.
Name the natural satellite of Earth.
Answer:
Moon.

Question 36.
Why a multi-stage rocket is required to launch a satellite?
Answer:
This is done to save fuel.

Question 37.
Mention one difference between g and G.
Answer:
The value of ‘G’ remains the same throughout the universe while the value of ‘g’ varies from place to place.

Question 38.
When an apple falls towards the Earth, the Earth moves up to meet the apple. Is the statement true? If yes, why is the Earth’s motion not noticeable?
Answer:
Yes, the statement is true. The acceleration of Earth is very small as compared to that of apple as the mass of Earth is very large.

Question 39.
Which of the following observations point to the equivalence of inertial and gravitational mass? Why?
(a) Two spheres of different masses dropped from the top of a long evacuated tube reach the bottom of the tube at the same time.
(b) The time period of a simple pendulum is independent of its mass.
(c) The gravitational force on a particle inside a hollow isolated sphere is zero.
(d) For a man in a closed cabin that is falling freely under gravity, gravity disappears.
(e) An astronaut inside a spaceship orbiting around the Earth feels weightless.
(f) Planets orbiting around the Sun obey Kepler’s Third Law.
(g) Gravitational force on a body due to the Earth is equal and opposite to the gravitational force on the Earth due to the body.
Answer:
As bodies are in motion in observations (a), (b), (d), (e), (f), thus, these point to the equivalence of inertial and gravitational masses.

Question 40.
How will the value of acceleration due to gravity be affected if the Earth begins to rotate at a speed greater than its present speed?
Answer:
Acceleration due to gravity will decrease if the angular speed of rotation of Earth increases.

Question 41.
How do we choose zero levels of gravitational potential energy?
Answer:
It corresponds to the infinite separation between two interacting masses.

Question 42.
Is it possible to put an artificial satellite in an orbit in such a way that it always remains visible directly over New Delhi? Why?
Answer:
It is not possible. This is because New Delhi is not in the equatorial plane.

Question 43.
A body is suspended with a spring balance attached to the ceiling of an elevator. The balance shows a reading of 5 divisions when the elevator is stationary. During the downward acceleration of the elevator, the balance shows zero reading. Do you think that the inertial mass and gravitational mass of the body are equal? Justify your answer.
Answer:
Yes, they will be equal. The two masses differ only when the velocity of the lift approaches the velocity of light.

Question 44.
Does a comet move faster at aphelion or perihelion?
Answer:
At the perihelion where it is close to Sun, the comet moves faster.

Question 45.
Due to some unforeseen event, the planet of the I0 satellites of Jupiter does not pass through the center of Jupiter. Will the orbit of I0 be stable? Why?
Answer:
No. For a stable orbit, the plane of the orbit must pass through the center (equatorial plane) of the planet.

Question 46.
Is it appropriate to describe the condition of weightlessness as the condition of masslessness? Why?
Answer:
No. Weight and mass are different physical quantities. A body may have zero weight but never zero mass. So it is not appropriate to describe the condition of weightlessness as a condition of masslessness.

Question 47.
Define central force.
Answer:
It is defined as the force which is always directed along the position vector of the point of application of the force w.r.t. the fixed point i.e. away or towards a fixed point.

Question 48.
The angular momentum is always conserved in the motion under a central force. Name the two results that follow from this.
Answer:

  1. The motion of a particle under the central force is always confined to a plane.
  2. The position vector of the particle w.r.t. the center of force has a constant areal velocity i.e. the position vector sweeps out equal areas in equal times as the particle moves under the influence of the central force.

Question 49.
Why an astronaut has a sense of weightlessness in a satellite revolving around the Earth?
Answer:
For revolving around the Earth, the astronaut and the satellite require the centripetal force, and their weight is used up in providing the necessary centripetal force. So the astronaut feels weightlessness in space.

Question 50.
Why an astronaut in an orbiting spacecraft is not in zero-gravity although he is in a state of weightlessness?
Answer:
We know that acceleration due to gravity depends on the height, so there is some value of acceleration due to gravity at every point on the orbit of the spacecraft and his weight is used up in providing the necessary centripetal force for the orbital motion.

Gravitation Important Extra Questions Short Answer Type

Question 1.
Explain how the weight of the body varies en route from the Earth to the moon. Would its mass change?
Answer:
When a body is taken from Earth to the moon, then its weight slowly decreases to zero and then increases till it becomes 16th of the weight of the body on the surface of the moon.

We know that mgh = mg (1 – 2hR)

As h increases, gh, and hence mgh, decreases. When R = R2 the force of attraction of Earth is equal to the force of attraction of the moon.

Then gh = 0, so mg becomes zero, and the value of g on the moon’s surface is 16th of its value on the surface of Earth. Hence on increasing h beyond R2, mg starts increasing due to the gravity of the moon. f ts mass remains constant.

Question 2.
Among the known type of forces in nature, the gravitational force ¡s the weakest. Why then does ¡t play a dominant role in the motion of bodies on the terrestrial, astronomical, and cosmological scale?
Answer:
Electrical forces are stronger than gravitational forces for a given distance, but they can be attractive as well as repulsive, unlike gravitational force which is always attractive. As a consequence, the forces between massive neutral bodies are predominantly gravitational and hence play a dominant role at long distances. The strong nuclear forces dominate only over a range of distances of the order of 10-14 m to 10-15m.

Question 3.
Show that the average life span of humans on a planet in terms of its natural years is 25 planet years if the average span of life on Earth is taken to be 70 years.
Answer:
Take the distance between Earth and Sun twice the distance between Earth and planet. According to Kepler’s third law of planetary motion,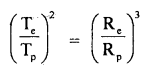
Class 11 Physics Important Questions Chapter 8 Gravitation 1
where Te, TR is the average life span on Earth and planet respectively.
Rg = distance between Earth and Sun.
Rp = distance between Earth and planet.
Here, Re = 2Rp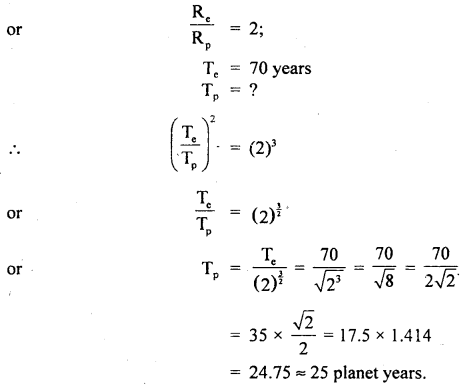
Class 11 Physics Important Questions Chapter 8 Gravitation 2

Question 4.
Hydrogen escapes faster from the Earth than oxygen. Why?
Answer:
The thermal speed of hydrogen is much larger than oxygen. Therefore a large number of hydrogen molecules are able to acquire escape velocity than that of oxygen molecules. Hence hydrogen escapes faster from the Earth than oxygen.

Question 5.
In a spaceship moving in a gravity-free region, the astronaut will not be able to distinguish between up and down. Explain why?
Answer:
The upward and downward sense is due to the gravitational force of attraction between the body and the earth. In a spaceship, the gravitational force is counterbalanced by the centripetal force needed by the satellite to move around the Earth in a circular orbit. Hence in the absence of zero force, the astronaut will not be able to distinguish between up and down.

Question 6.
Why the space rockets are generally launched from west to east?
Answer:
Since the Earth revolves from west to east around the Sun, so when the rocket is launched from west to east, the relative velocity of the rocket = launching velocity of rocket + linear velocity of Earth. Thus the velocity of the rocket increases which helps it to rise without much consumption of the fuel. Also, the linear velocity of Earth is maximum in the equatorial plane.

Question 7.
Explain why the weight of a body becomes zero at the center of Earth.
Answer:
We know that the weight of a body at a place below Earth’s surface is given by
W = mgd …. (i)
Where gd = acceleration due to gravity at a place at a depth ‘d’ below Earth’s surface and is given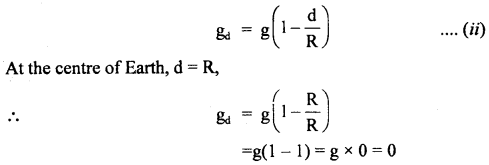
Class 11 Physics Important Questions Chapter 8 Gravitation 3
From Eqn. (i) W = 0 at the center of Earth.
i.e., g decreased with depth and hence becomes zero at the center of Earth, so W = 0 at Earth’s center.

Question 8.
We cannot move even our little fingers without disturbing the whole universe. Explain why.
Answer:
According to Newton’s law of gravitation, every particle of this universe attracts every other particle with a force that is inversely proportional to the square of the distance between them. When we move our fingers, the distance between the particles changes, and hence the force of attraction changes which in turn disturbs the whole universe.

Question 9.
Explain why tennis ball bounces higher on hills than on plains.
Answer:
The value of acceleration due to gravity is lesser on hills than on the plains, so the weight of the tennis ball at hills is lesser than that on the plains and hence it re-bounces more. In other words, the force with which the Earth attracts the ball on hills will be lesser than that on the plains.

Question 10.
Why is the atmosphere comparatively rarer on some of the planets as compared to that on earth?
Answer:
The escape velocity of some of the planets is very small as compared to that on the surface of Earth. Most of these gases have their root mean square (r.m.s.) velocities more than the escape velocity on these planets and hence they have escaped from the surface of these planets and hence the atmosphere is rarer on some of the planets than on Earth.

Question 11.
Why moon has no atmosphere? Explain.
Answer:
An atmosphere means the presence of a mixture of a number of gases. The molecules of these gases are in the state of continuous random motion moving with different velocities. As the value of escape velocity On the surface of the moon is small (only 2.5 km s-1), the molecules of gases with velocities greater than the escape velocity moved out of the atmosphere. As time passed, nearly all the molecules escaped from the moon’s atmosphere.

Question 12.
Under what conditions a satellite will be called geo-stationary?
Answer:
The following are the conditions to be fulfilled by a satellite to be geostationary:

  1. The time period of the satellite around the Earth must be equal to the rotational period of the satellite i.e. 24 hours.
  2. The direction of motion of the satellite must be the same as that of the Earth.
  3. The height of the geostationary satellite must be about 36000 km.

Question 13.
What are the uses of Artificial Satellites?
Answer:
Following are some of the important uses of Artificial Satellites:

  1. They are used as communication satellites to send messages to distant places.
  2. They are used as weather satellites to forecast weather.
  3. They are used to know the exact shape of Earth.
  4. They are used to telecast T.V. programs to distant places.
  5. They are used to explore the upper region of the atmosphere.

Question 14.
Under what condition, a rocket fired from Earth will launch an artificial satellite around Earth?
Answer:
Following are the basic conditions:

  1. The rocket must take the satellite to a suitable height above the surface of Earth.
  2. From the desired height, the satellite must be projected with a suitable velocity called orbital velocity.
  3. In the orbital path of the satellite, the air resistance should be negligible so that its velocity does not decrease and it does not bum due to the heat produced.

Question 15.
The acceleration due to gravity on a planet is 1.96 ms-2. If it is safe to jump from a height of 2m on the Earth, what will be the corresponding safe height on the planet?
Answer:
The safety of a person depends upon the momentum with which the person hits the planet. Since the mass of the person is constant therefore the maximum velocity (v) is the limiting factor.
In case of earth, v2 = 2gehe …. (i)
In case of planet, v2 = 2gphp …. (ii)
where he, hp are safe heights of jumping from Earth and planet respectively,
Here, he = 2m; gp = 1.96 ms-2, ge = 9.8 ms-2

∴ From (i) and (ii), we get
2ge he = 2gp hp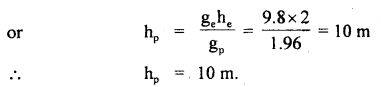
Class 11 Physics Important Questions Chapter 8 Gravitation 4

Question 16.
Is it correct to state that we are living at the bottom of a gravitational well? Why?
Answer:
Yes. The gravitational force varies with distance from the center of Earth as shown in the fig. below. The graph clearly shows a minimum at a point on the surface of Earth. Thus, it is correct to state that we are living at the bottom of a gravitational well.
Class 11 Physics Important Questions Chapter 8 Gravitation 5

Question 17.
Prove that if two spheres of the same material, mass, and radius, are put in contact, the gravitational attraction between them is directly proportional to the fourth power of their radius.
Answer:
Let m = mass of each sphere.
R = radius of each sphere,
p = density of the material of the spheres.
∴ If F be the gravitational attraction between them, then according to Newton’s law of gravitation,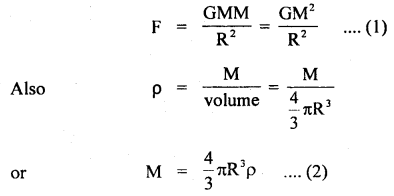
Class 11 Physics Important Questions Chapter 8 Gravitation 6
Class 11 Physics Important Questions Chapter 8 Gravitation 7
∴ From (1) and (2), we get
Class 11 Physics Important Questions Chapter 8 Gravitation 8

Question 18.
Prove that if the gravitational attraction of the Sun on the planets varies as nth power of the distance (of the planet from the Sun), then a year of the planet will be proportional to R(n + 1)/2.
Answer:
Let m = mass of the planet
R = radius of its orbit around Sun
M = mass of the sun
V = orbital speed of the planet
Then,
Class 11 Physics Important Questions Chapter 8 Gravitation 9

Question 19.
The figure below shows four arrangements of three particles of equal masses: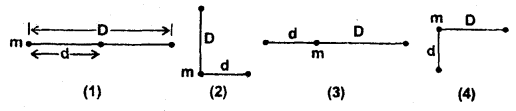
Class 11 Physics Important Questions Chapter 8 Gravitation 10
(a) Rank the arrangements according to the magnitude of the net gravitational force on the particle labeled m, greatest first, and explain.
(b) In arrangement 2, in the direction of the net force closer to the line of length d or to the line of length D?
Answer:
(a) 1,2 and 4 tie, 3 (b) line d.
Explanation: (a) An arrangement (1), the forces exerted by both the particles are in the same direction. So these forces get add up to give maximum force. In arrangements (2) and (4), the angle between individual forces as well as the distances are the same. So the magnitudes of the net forces in (2) and (4) are the same and greater than in arrangement (3) and less than in (1).

In arrangement (3), the angle between the separate forces is maximum i.e. 180. So the net force is minimum.

(b) Due to the smaller distance, force is greater the net force always makes a smaller angle with the larger force as compared to the angle with a smaller force.

Question 20.
Why the atmosphere of Jupiter contains light gases (generally hydrogen) whereas the Earth’s atmosphere has little hydrogen gas?
Answer:
The escape velocity of Jupiter is much larger than the escape velocity of Earth. So to escape from the surface of Jupiter, a very large velocity is required. Since the thermal velocity of hydrogen gas molecules is lesser than the escape velocity of Jupiter, therefore hydrogen can’t escape from the surface of Jupiter.

Question 21.
Why does not an astronaut use a simple pendulum clock in a satellite revolving around the Earth?
Answer:
Acceleration due to gravity is zero inside a satellite therefore the time period of vibration of the simple pendulum
T = 2π lg−−√ will be infinity

So the pendulum will do not vibrate inside the satellite and hence the clock will not work. So astronaut does not use a simple pendulum clock in a satellite.

Question 22.
Show that Kepler’s Second law is the law of conservation of angular momentum.
Answer:
The second law states that the areal velocity is constant i.e. area covered by the radius vector is the same in equal intervals of time. If the velocity and radius at the time, t1 is v1 and r1 while at another place these are v2 and r2 in the same time, then the area covered by the planet in these intervals are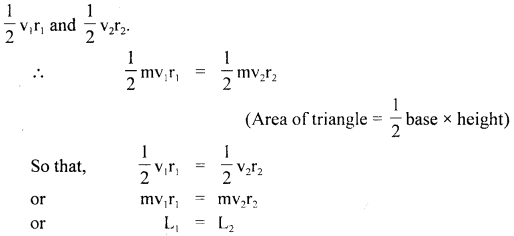
Class 11 Physics Important Questions Chapter 8 Gravitation 11
This shows that the law leads to the conservation of angular momentum law.

Question 23.
Astronomical observations show that Mercury moves faster and Pluto slower, why is it so?
Answer:
Planets obey Kepler’s Third law of motion.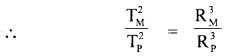
Class 11 Physics Important Questions Chapter 8 Gravitation 12
TM = time of revolution of Mercury around Sun at distance RM.
TP = time of revolution of Pluto around Sun at distance RP.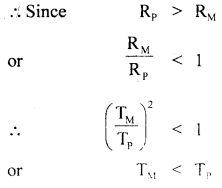
Class 11 Physics Important Questions Chapter 8 Gravitation 13
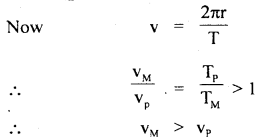
Class 11 Physics Important Questions Chapter 8 Gravitation 14
Pluto moves slower as compared to Mercury due to the reason that RP > RM.

Question 24.
Calculate the density of Earth.
Answer:
Let us assume the Earth to be a homogeneous sphere of radius R, density ρ, and mass M.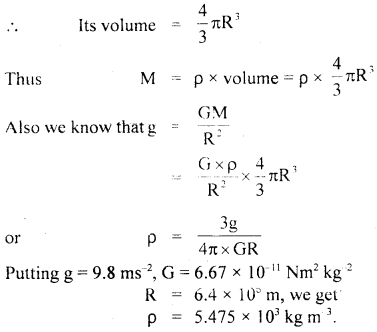
Class 11 Physics Important Questions Chapter 8 Gravitation 15

Question 25.
Find an expression for the torque on a body due to gravity. Show that this torque about the center of mass of a body is zero.
Answer:
Suppose a body is made up of a large number of particles of masses m1, m2, m3, ….. etc. situated at r1, r2, r3,… etc. positions from the center of mass of the body. Each particle is pulled by gravity with a force F = mg. Let this force on ith particle be f = mg. Since the position vector of this particle is r1, hence the torque on this particle due to a national poll of the Earth is
τi = ri × mig
Then total torque on the body
Class 11 Physics Important Questions Chapter 8 Gravitation 16
Then torque about the center of mass is eventually 0 as rcm = 0 from the center of mass,
∴ τ = 0.

Question 26.
How do Kepler’s laws lead to Newton’s universal law of gravitation?
Answer:
Kepler’s laws are applicable to the motion of planets around the Sun. Almost all planets revolve around the Sun in nearly circular orbits.

Let m = mass of a planet.
M = mass of Sun.
r = radius of the circular orbit of the planet, around the Sun.
v = linear velocity of the planet in its orbit.
T = Time period of the planet.

The centripetal force required by the planet is.
F = mv2r
But v = circumference of the orbit / period of revolution = 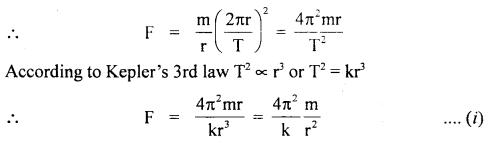2πrT
Class 11 Physics Important Questions Chapter 8 Gravitation 17
This centripetal force is provided by the force of attraction between the Sun and the planet. According to Newton, the force of attraction between the planet and the Sun is mutual.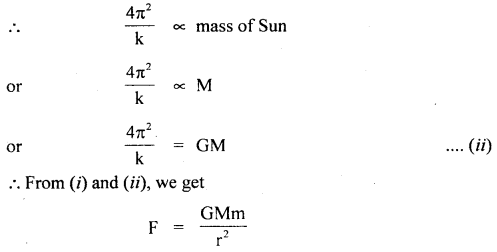
Class 11 Physics Important Questions Chapter 8 Gravitation 18
which is Newton’s law of gravitation.

Question 27.
Explain how the mass of the Sun can be determined by studying the motion of Earth around it.
Answer:
The Sun’s gravitational force provides the necessary centripetal force to the Earth for its revolution around the Sun in an orbit of radius r. Let the mass of Sun be Ms and that of Earth Mc. Thus centripetal force is then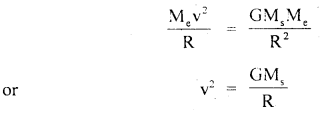
Class 11 Physics Important Questions Chapter 8 Gravitation 19
where R = radius of orbit of Earth.
Let T = Time period of revolution of Earth around Sun, then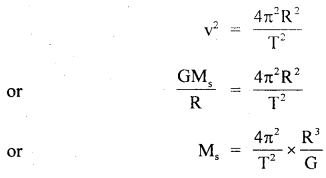
Class 11 Physics Important Questions Chapter 8 Gravitation 20
we know R, G, and T so the mass of the Sun can be found out by putting their values.

Question 28.
Derive an expression for the gravitational potential energy above the surface of Earth.
Answer:
Let the body of mass m be taken at a height h above the surface of Earth. At any instant of time t, it reaches at a distance x from the center of Earth. The work done in raising the body through dx is
Class 11 Physics Important Questions Chapter 8 Gravitation 21
Hence, the work done in taking the body from the surface of the Earth (x = R) to a height h above the earth’s surface (x = R + h) is given by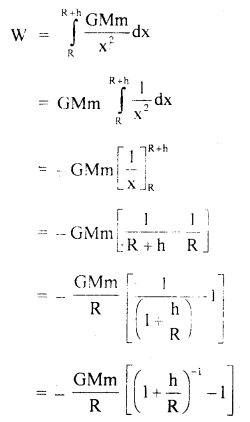
Class 11 Physics Important Questions Chapter 8 Gravitation 22
Class 11 Physics Important Questions Chapter 8 Gravitation 23
This work done is stored in the body in the form of gravitational
P.E. i.e. P.E. = W = mgh
P.E. above the surface of Earth = mgh.

Question 29.
What is the binding energy of the satellite?
Answer:
The minimum energy required to free a satellite from the gravitational attraction is called its binding energy. The binding energy is the negative value of the total energy of the satellite. Let a satellite of mass m be revolving around the earth of mass M and radius R.

∴ The total energy of satellite = P.E. + K.E.
Class 11 Physics Important Questions Chapter 8 Gravitation 24
Bindind energy of satellite = – (total energy of satellite)
GMm2R

Question 30.
In a cavendish experiment, two spheres of mass 10 g each are suspended with a torsion rod of length 2m when two lead spheres of mass 10 mg each are brought near the suspended sphere as shown in the figure, the spheres are displaced through 2mm each. The torsional rod is deflected through an angle of 0.02 radian. Calculate the value of G if the torsional couple per unit twist of the suspension wire is 1.66 × 10-4 Nm.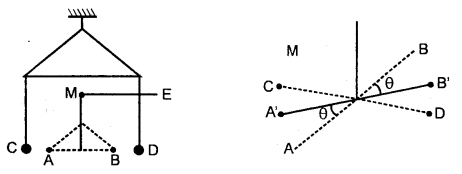
Class 11 Physics Important Questions Chapter 8 Gravitation 25
Answer:
Answer:
Force exerted by a bigger sphere on the small sphere is
F= GMm2R
where r = separation between bigger and small spheres.
M = mass of the bigger sphere.
m = mass of the smaller sphere.

The force on the two sides produces torque and the suspension wire produces opposite torque = cθ = restoring couple produced.
where c = Torsional couple per unit twist.
θ = Twist

If l be the length of torsion rod, the deflecting couple
= Fl = GMmR2 l

Since the rod is in equilibrium after the deflection
∴ deflecting couple = restoring couple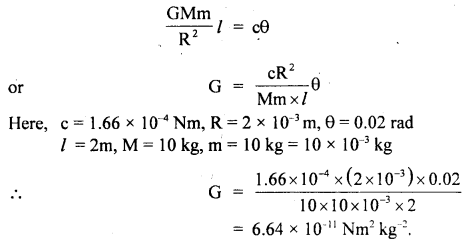
Class 11 Physics Important Questions Chapter 8 Gravitation 26

Gravitation Important Extra Questions Long Answer Type

Question 1.
(a) Derive the expression for the orbital velocity of an artificial Earth’s satellite. Also, derive its value for an orbit near Earth’s surface.
Answer:
Let m = mass of the satellite.
M, R = mass and radius of Earth.
h = height of the satellite above the surface of Earth.
r = radius of the robot of the satellite
= R + h.
v0 = orbital velocity of the satellite.

The centripetal force 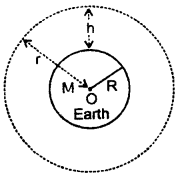mv20r required by the satellite to move in a circular orbit is proved by the gravitational force between satellite and the Earth.
Class 11 Physics Important Questions Chapter 8 Gravitation 27
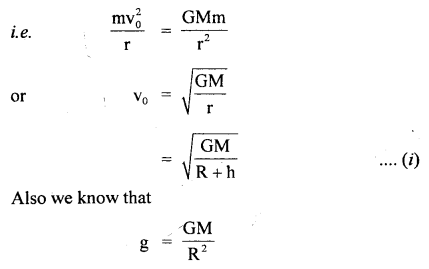
Class 11 Physics Important Questions Chapter 8 Gravitation 28
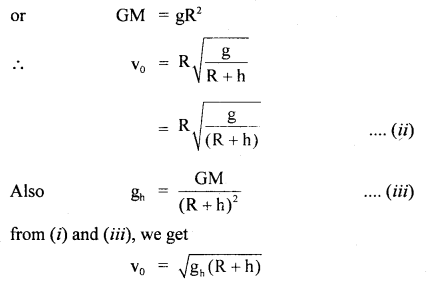
Class 11 Physics Important Questions Chapter 8 Gravitation 29
If the satellite is close to the earth’s surface, then h ≈ 0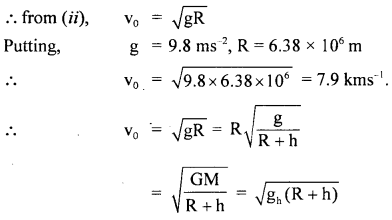
Class 11 Physics Important Questions Chapter 8 Gravitation 30
(b) Derive the expression for escape velocity of a body from the surface of Earth and show that it 2–√ times the orbital velocity close to the surface of the Earth. Derive its value for Earth.
Answer:
Escape velocity is the minimum velocity with which a body is projected from Earth’s surface so as to just escape its gravitational pull or of any other planet. It is denoted by ve.

Expression: Consider the earth to be a homogenous sphere of radius R, mass M, center O, and density p.
Let m = mass of the body projected from point A on the surface of Earth with vel. ve.

∴ K.E. of the body at point A = 12 mve2 …(i)
Let it reaches a point P at a distance x from O. If F be the gravitational force of attraction on the body at P, then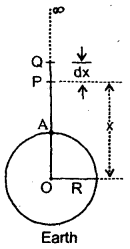
Class 11 Physics Important Questions Chapter 8 Gravitation 31
F = GMmx2 …(ii)

Let it further moves to Q by a distance dx.
If dW be the work done in moving from P to Q, then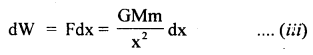
Class 11 Physics Important Questions Chapter 8 Gravitation 32
If w be the total work done in moving the body from A to ∞,
Then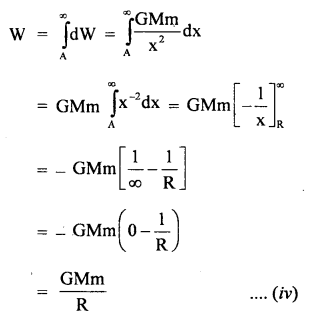
Class 11 Physics Important Questions Chapter 8 Gravitation 33
∴ According to the law of conservation of energy
K.E. = RE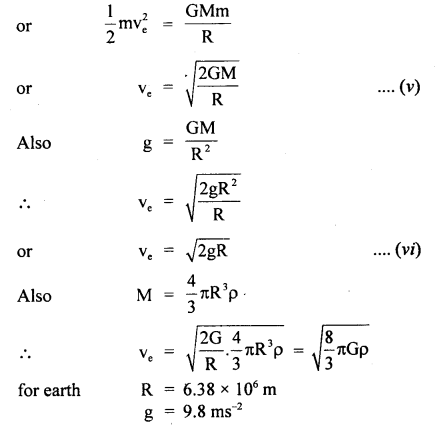
Class 11 Physics Important Questions Chapter 8 Gravitation 34
Class 11 Physics Important Questions Chapter 8 Gravitation 35
Relation between ve and vo: Also we know that the orbital velocity around Earth close to its surface is given
by v0 = gR−−−√
and ve = 2gR−−−−√ = 2–√ gR−−−√
= 2–√v0
Hence proved.

Question 2.
(a) Explain Newton’s law of gravitation.
Answer:
We know that Newton’s law of gravitation is expressed mathematically as: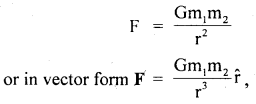
Class 11 Physics Important Questions Chapter 8 Gravitation 36
where r̂ = unit vector along F
It was found that law is equally applicable anywhere in the universe between small and big objects like stars and galaxies. The value of G remains the same everywhere. (Some scientists have claimed that as the size of the object under consideration becomes big like a galaxy, the value of G also changes). Hence this law of Newton is also called Newton’s universal law of gravitation.

The force of attraction is called the force of gravitation or gravitational force. This force is only attractive and is never repulsive. The force is both ways i.e., particle 1 attracts particle 2 and so does particle 2 attracts particle 1.
Hence F12 = – F21.

The law is a direct outcome of the study of acceleration of bodies. Newton wondered how Moon revolves around the Earth or other planets revolve around the Sun. His calculations showed that the Moon is accelerated by the same amount as does any other object towards the Earth.

His famous narration of the apple falling from the tree and noticing every other object fall towards Earth led to the announcement of his famous law of gravitation about 50 years later in his book ‘Principia’.

Out of the known forces in nature, the Gravitational force is the weakest, yet it is the most apparent one as it acts for long distances and between objects which are visible to us. The law of gravitation has been used to determine the mass of heavenly bodies. It has been used to study the atmosphere of planets. Man-made satellites remain in the orbits due to gravitation.

(b) Define gravitational field intensity. Derive its expression at a point at a distance x from the center of Earth. How is it related to acceleration due to gravity?
Answer:
The gravitational field intensity at a point is defined as the force acting on a unit mass placed at that point in the field.

Thus, the gravitational field intensity is given by:
E = Fm
Now at distance x from the center of Earth, the gravitational force is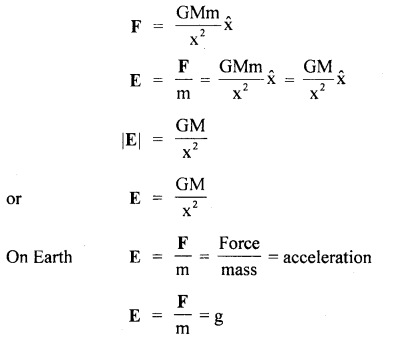
Class 11 Physics Important Questions Chapter 8 Gravitation 37
So, the intensity of the gravitational field at the surface of Earth is equal to the acceleration du&to gravity.

Question 3.
Discuss the variation of acceleration due to gravity with:
(a) Altitude or height
(b) Depth
(c) Latitude i.e. due to rotation of Earth.
Answer:
Let M, R be the mass and radius of the earth with center O.
g = acceleration due to gravity at a point
An on Earth’s surface.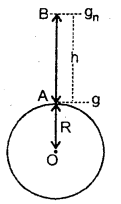
Class 11 Physics Important Questions Chapter 8 Gravitation 38
(a) Variation of g with height: Let g0h be the acceleration due to gravity at a point B at a height h above the earth’s surface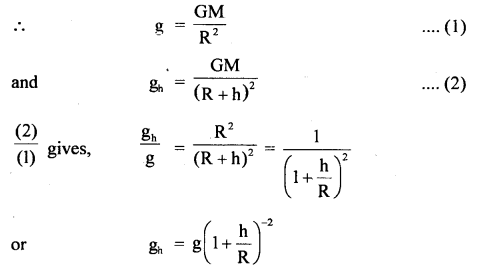
Class 11 Physics Important Questions Chapter 8 Gravitation 39

If h << R, then using Binomial Expansion, we get
Class 11 Physics Important Questions Chapter 8 Gravitation 40
Thus, from Eqn. (3), we conclude that acceleration due to gravity decreases with height.

(b) With depth: Let the Earth be a uniform sphere.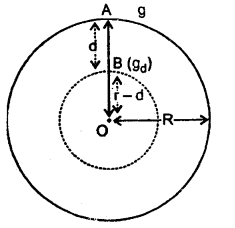
Class 11 Physics Important Questions Chapter 8 Gravitation 41
Let gd = acceleration due to gravity at a depth d below earth’s surface i.e. at point B.

Let ρ = density of Earth of mass M.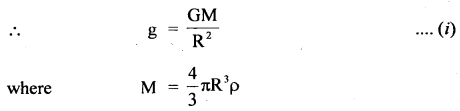
Class 11 Physics Important Questions Chapter 8 Gravitation 42
Also, let M’ = mass of Earth at a depth d, then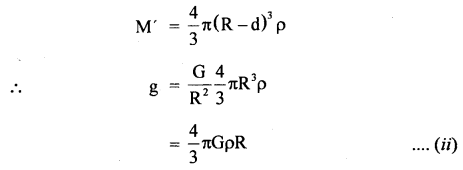
Class 11 Physics Important Questions Chapter 8 Gravitation 43
Class 11 Physics Important Questions Chapter 8 Gravitation 44

From equation (iv), we see that acceleration due to gravity decreases with depth.
Special case: At the center of Earth, d = R
∴ gd = 0

Hence an object at the center of Earth is in a state of weightlessness.

(c) Variation of g with latitude:
Let m = mass of a particle at a place P of latitude X.
ω = angular speed of Earth about axis NS.
Class 11 Physics Important Questions Chapter 8 Gravitation 45

As the earth rotates about the NS axis, the particle at P also rotates and describes a horizontal circle of radius r,
where r = PC = OP cos λ, = R cos λ

Let g’ be the acceleration due to gravity at P when the rotation of Earth is taken into account. Now due to the rotation of the earth, two forces that act on the particle at P are:

  1. Its weight mg, acting along with PO.
  2. Centrifugal force mroo2 along PO’.

∴ The angle between them = 180 – λ
∴ According to the parallelogram law of vector addition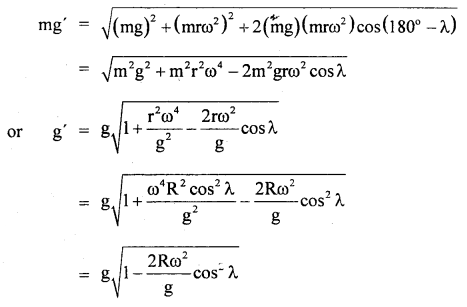
Clas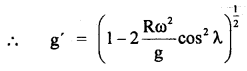s 11 Physics Important Questions Chapter 8 Gravitation 46
[As Rω2 g is very small (= 1289)so its square and higher powers are neglected.]
Class 11 Physics Important Questions Chapter 8 Gravitation 47
Using binomial expansion, we get
Class 11 Physics Important Questions Chapter 8 Gravitation 48
⇒ g decreases with the rotation of the earth.

  1. At poles, λ = 90°, ∴ g’ = gp = g
  2. At equator, λ = 0, g’ = ge = g- Rω2.

Clearly gp > ge.

Numerical Problems:

Question 1.
At what height from the surface of Earth will the value of g be reduced by 36% from the value at the surface? The radius of Earth, R = 6400 km.
Answer:
Let h be the height above the surface at which g reduces by 36% i.e. becomes 64% of that at the surface i.e.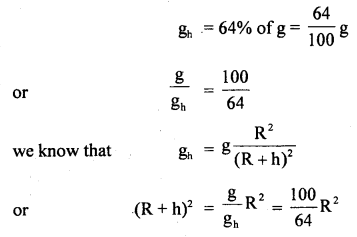
Class 11 Physics Important Questions Chapter 8 Gravitation 49
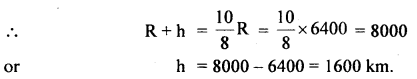
Class 11 Physics Important Questions Chapter 8 Gravitation 50

Question 2.
A mass of 5 kg is weighed on a balance at the top of a tower 20 m high. The mass is then suspended from the pan of the balance by a fine wire 20 m long and is reweighed. Find the change in the weight, R = 6400 km.
Answer:
h = 20 m
m = mass = 5 kg
R = 6400 km = 64 × 105m
g = 9.8 ms-2

As h < R, so from relation,
Class 11 Physics Important Questions Chapter 8 Gravitation 51
Now weight at the foot of tower, W = mg
Now weight at the top of tower, Wh = mgh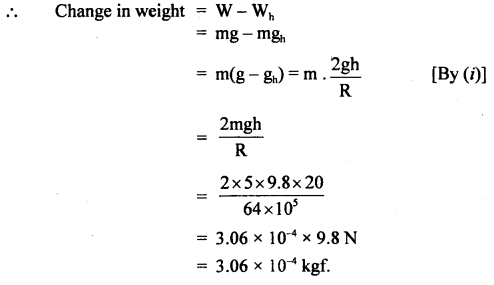
Class 11 Physics Important Questions Chapter 8 Gravitation 52

Question 3.
How much faster than its present rate should the Earth rotate about its axis in order that the weight of the body on the equator may be zero? What will be the duration of the day? The radius of Earth, R = 6400 km.
Answer:
We know that the value of ‘g’ at latitude λ is
gλ = g – R ω2 cos2λ
at equator λ = 0,
∴ cos 0 = 1
∴ gλ = g – Rω2

Let ω1 = new angular speed of rotation so that weight at equator becomes zero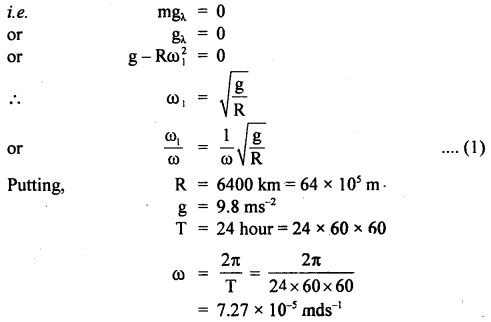
Class 11 Physics Important Questions Chapter 8 Gravitation 53
in equation (1), we get
Class 11 Physics Important Questions Chapter 8 Gravitation 54
i.e. the Earth must rotate 17 times faster thair its present speed. Now the duration of the day 24 hours i.e. earth makes one rotation in 24 hours. When it rotates 17 times faster, it will make 17 rotations in 24 hours

∴ Time for 1 rotation (Duration of Day) = 2417 = 1.412 hours.

Question 4.
Show the moon would escape if its speed were increased by 42%.
Answer:
Let M = mass of Earth.
m = mass of the moon,
r = radius of the circular orbit of the moon around the Earth.
vo = orbital velocity of the moon.

The centripetal force needed by moon = Gravitational force between the Earth and moon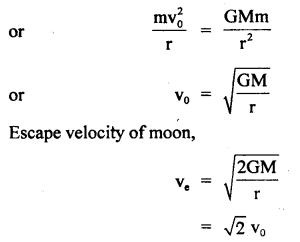
Class 11 Physics Important Questions Chapter 8 Gravitation 55
∴ % increase in the velocity of the moon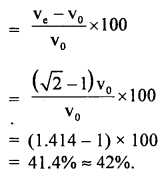
Class 11 Physics Important Questions Chapter 8 Gravitation 56

Question 5.
If the Earth has a mass 9 times and a radius twice of the planet Mars, calculate the minimum velocity required by a rocket to pull out of the gravitational force of Mars. Escape velocity on the surface of Earth is 11.2 km s-1.
Answer:
Let Mm and be the mass and radius of Mars,
and M, R = mass and radius of Earth.

Also, let v and Vm be the escape velocities from Earth and Mars respectively.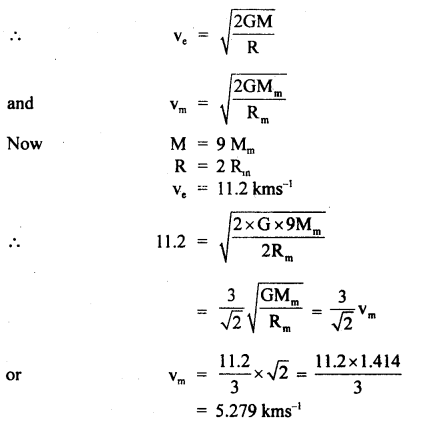
Class 11 Physics Important Questions Chapter 8 Gravitation 57

Question 6.
What would be the duration of the year ¡f the distance between the Earth and the Sun gets doubled?
Answer:
Let T1 = time period of Earth about the Sun when the distance between Sun and Earth is R and T2 = time period of Earth about the Sun when the distance between Earth and Sun is 2R.

Now using the relation,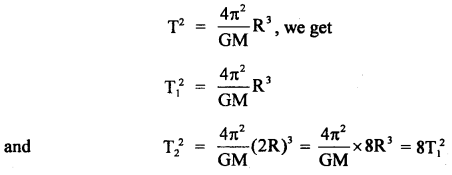
Class 11 Physics Important Questions Chapter 8 Gravitation 58
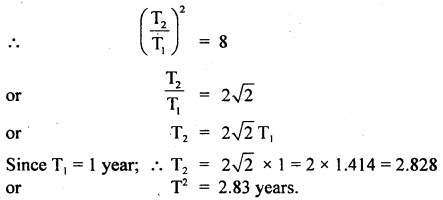
Class 11 Physics Important Questions Chapter 8 Gravitation 59

Question 7.
The period of the moon around Earth is 27.3 days and the radius of its orbit is 3.9 × 105 km. Find the mass of Earth, G = 6.67 × 10-11 Nm2 Kg-2.
Answer:
T = time period = 27.3 days
= 27.3 × 24 × 60 × 60s.

r = radius of orbit
= 3.9 × 105 km = 3.9 × 108m.

G = 6.67 × 10-11 N m2 kg-2.
M = mass of Earth = ?

Let m, v be the mass and linear velocity of the moon. Then the centripetal force to the moon is provided by the force of attraction between Earth and the moon.
Class 11 Physics Important Questions Chapter 8 Gravitation 60

Question 8.
Find the percentage decrease in weight of a body when taken 16 km below the surface of Earth. Take the radius of Earth = 6400 km.
Answer:
Here, R = 6400 km, d = 16km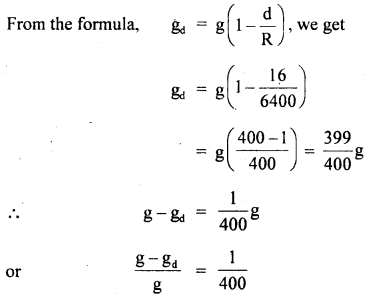
Class 11 Physics Important Questions Chapter 8 Gravitation 61
If m = mass of the body, then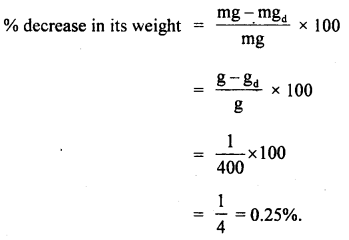
Class 11 Physics Important Questions Chapter 8 Gravitation 62

Question 9.
A mass M is broken into two parts m and M – m. How are m and M related so that the force of gravitational attraction between the two parts is maximum?
Answer:
Let r = distance between two parts i.e. m and M – m
If F be the gravitational attraction between m and M – m, then from Newton’s law of gravitation,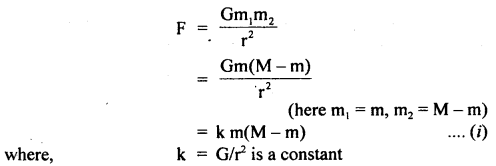
Class 11 Physics Important Questions Chapter 8 Gravitation 63
eqn. (i) may be rewritten as: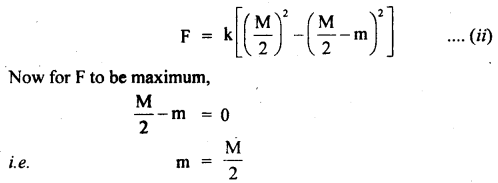
Class 11 Physics Important Questions Chapter 8 Gravitation 64
∴ each piece has equal mass.

Aliter:
For F to be maximum,
Class 11 Physics Important Questions Chapter 8 Gravitation 65

Question 10.
A satellite orbits the Earth at a height of 500 km from its surface.
Calculate (i) K.E.
(ii) P.E.
(iii) Total energy.
Mass of satellite is 300 kg, mass of Earth is 6 × 1024 kg, radius of Earth = 6.4 × 106 m, G = 6.67 × 10-11 Nm2 kg-2. Will your answer alter if the Earth were to shrink suddenly to half its size?
Answer: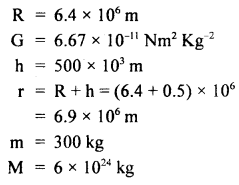
Class 11 Physics Important Questions Chapter 8 Gravitation 66
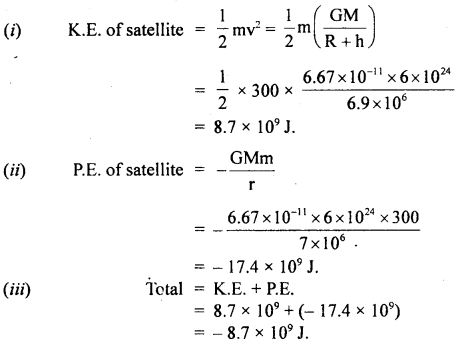
Class 11 Physics Important Questions Chapter 8 Gravitation 67
If the earth shrinks suddenly to half its radius (R becomes R/2) but r = (R + h) remains unchanged, then the answer will not alter.

Question 11.
If a body is projected with a velocity v greater than ve (escape velocity from Earth’s surface), find its velocity in interstellar space.
Answer:
Let v’ be the velocity of the body of mass m in interstellar space where the gravitational field of Earth is zero; so the P.E. of the body is also zero.

∴ According to the law of conservation of energy,
we have, K.E. + RE. at the surface of Earth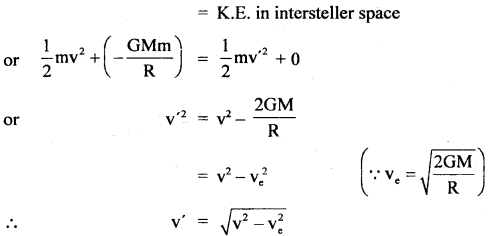
Class 11 Physics Important Questions Chapter 8 Gravitation 68

Question 12.
What will be the acceleration due to gravity on the surface of the moon if its radius is 1/4th of the radius of Earth and its mass l/80th of the mass of Earth?
Answer:The value of acceleration due to gravity is g =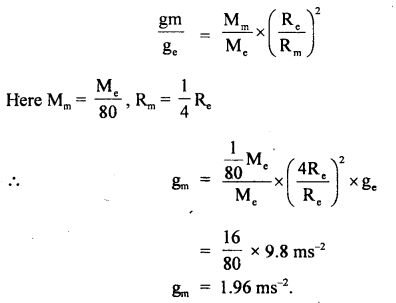GMR2
Class 11 Physics Important Questions Chapter 8 Gravitation 69

Question 13.
Consider an Earth satellite so positioned that it appears stationary to an observer on Earth and serves the purpose of a fixed relay station for intercontinental transmission of television and other communications. What should be the height at which the satellite should be positioned and what would be the direction of its motion? (Radius of Earth R = 6400 km.)
Answer:
Suppose the satellite is stationed at height h from the surface of the Earth, then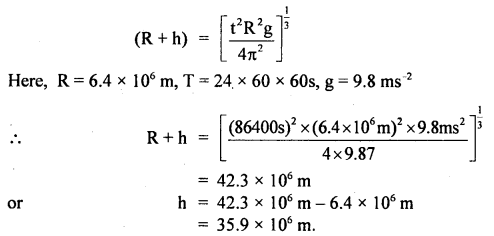
Class 11 Physics Important Questions Chapter 8 Gravitation 70

Question 14.
If the moon followed a circular orbit of radius r around the Earth with a uniform angular velocity co, so that ω2r2 = gR2, where R is the radius of the Earth and g the acceleration due to gravity. If r = 60R and the period of revolution of the moon around the Earth is 27.3 days. Find R.
Answer: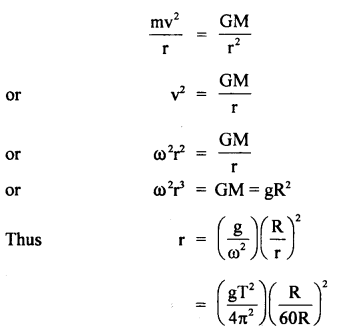
Class 11 Physics Important Questions Chapter 8 Gravitation 71
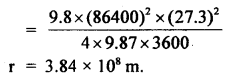
Class 11 Physics Important Questions Chapter 8 Gravitation 72

Question 15.
The mass of asteroid Ceres is approximately 7 × 1020 kg and its diameter is 1100 km. What is the value of acceleration due to gravity at its surface? What would be the weight of an 80 kg astronaut on this asteroid?
Answer:
The radius of the asteroid Ceres,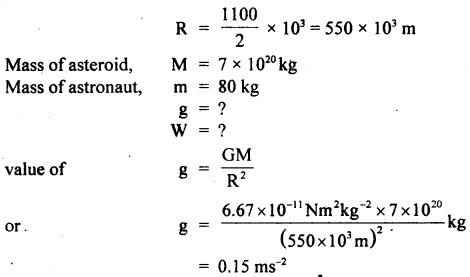
Class 11 Physics Important Questions Chapter 8 Gravitation 73
∴ Weight of astronaut, W = mg = 80 × 0.15 ms-2 = 12 N.

Question 16.
The mass of the Sun is 2 × 1030 kg and its radius 7 × 108 m. Calculate the rate of emission of radiation from it if its radius shrinks at the rate of 1 × 104 ms-1.
Answer:
The self-energy of the Sun, considering it spherical in shape, is given by
Class 11 Physics Important Questions Chapter 8 Gravitation 74
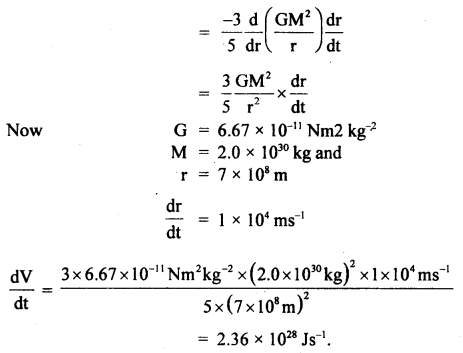
Class 11 Physics Important Questions Chapter 8 Gravitation 75

Question 17.
Two stars of mass 3 × 1031 kg each, in a double star, rotate about their common center of mass 1011 m part
(i) Calculate their common angular speed.
Answer:
Let one-star move in a circular orbit of radius R, then gravitational pull provides the necessary centripetal force. Also, the distance between two stars = x, and they have equal mass then x = 2R.
Therefore,
Class 11 Physics Important Questions Chapter 8 Gravitation 76
Substituting the given values, we have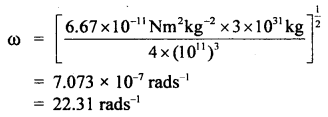
Class 11 Physics Important Questions Chapter 8 Gravitation 77

(ii) If a meteorite passes through its center of mass moving at right angles to the line joining the stars. In order to escape from the gravitational field of the double star what should be the speed of the meteorite?
Answer:
The meteorite can escape from the gravitational field if the sum of its K.E. and P.E. due to the two stars is greater than 0 i.e.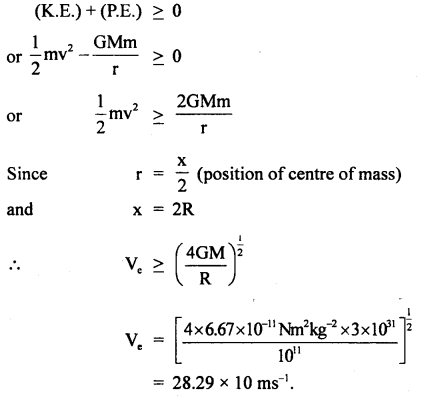
Class 11 Physics Important Questions Chapter 8 Gravitation 78

Question 18.
Neglecting the presence of other planets and satellites, calculate the binding energy of the Sun-Earth system. Mass of Earth ME = 6 × 1024 kg, mass of Sun = 1.98 × 1030 kg, Orbital radius of Earth R= 1.5 × 1011 m.
Answer:
The binding energy is equal to the amount of energy spent in bringing the Earth-Sun system from infinity to the distance R. In other words, this is equal to the energy required to separate them to infinity hence it is equal to (P.E.)g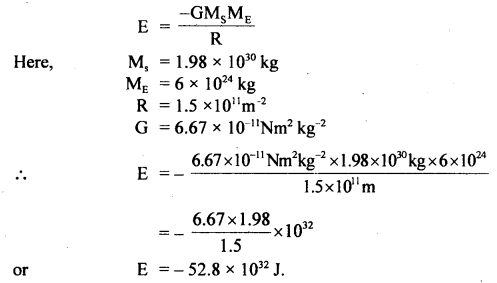
Class 11 Physics Important Questions Chapter 8 Gravitation 79

Question 19.
If the radius of the Earth decreases by 10%, the mass remaining unchanged, what will happen to the acceleration due to gravity?
Answer:
Here, let M, R be the mass and radius of the earth. If g be the acce. to due to gravity on earth’s surface, then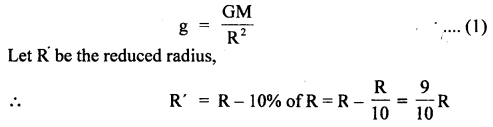
Class 11 Physics Important Questions Chapter 8 Gravitation 80
If g’ be the new value of acceleration.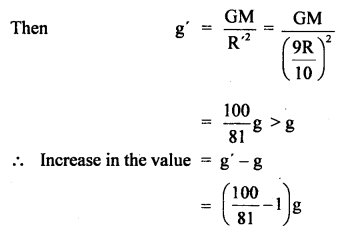
Class 11 Physics Important Questions Chapter 8 Gravitation 81
Class 11 Physics Important Questions Chapter 8 Gravitation 82

Question 20.
The ratio of the radii of the planets P1 and P2 is k and the ratio of the acceleration due to gravity on them is r. Calculate the ratio of the escape velocities from them.
Answer:
Let r1 and r2 be the radii of the planet P1 and P2 respectively having respective accelerations due to gravity g1, and g2.
Class 11 Physics Important Questions Chapter 8 Gravitation 83
If v1 and v2 be the escape velocities from P1 and P2 respectively, then using the relation,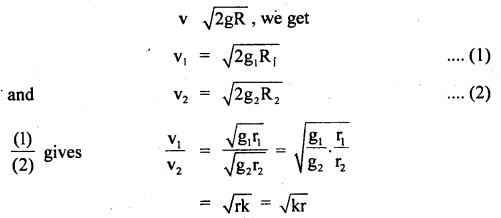
Class 11 Physics Important Questions Chapter 8 Gravitation 84

Question 21.
Calculate the temperature at which oxygen molecules can escape from the surface of Earth. Assume the radius of Earth as 6.4 × 106 m and g = 9.8 ms-2, the universal gas constant, R = 8.4 J mol-1 k-1.
Answer:
Here, Re = radius of Earth = 6.4 × 106 m
If a ve be the escape velocity of oxygen molecules from Earth’s surface, then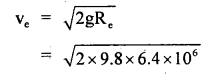
Class 11 Physics Important Questions Chapter 8 Gravitation 85
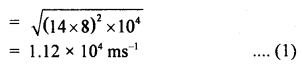
Class 11 Physics Important Questions Chapter 8 Gravitation 86
According to the kinetic theory of gases, the most probable velocity of the molecules of a gas is given by,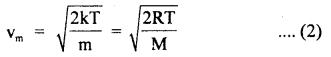
Class 11 Physics Important Questions Chapter 8 Gravitation 87
where M = molecular weight of the gas = 32 × 10-3 kg for O2.
R = 8.4J mol-1 k-1
For the escape of the gas molecules,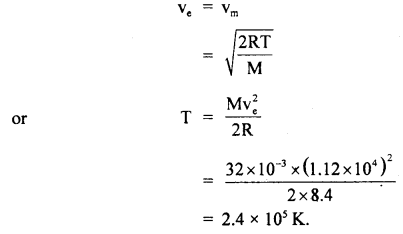
Class 11 Physics Important Questions Chapter 8 Gravitation 88

Question 22.
Find the gravitational force of attraction on a uniform rod of length L and mass m due to uniform earth as shown in Fig. How the rod will behave if L << r.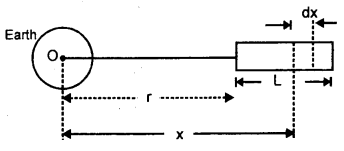
Class 11 Physics Important Questions Chapter 8 Gravitation 89
Answer:
Let M, R be the mass and radius of earth respectively. As the earth is uniform, so its whole mass may be supposed to be concentrated at its center O.

Let dF be the force due to earth on the mass element dx at a distance x from O.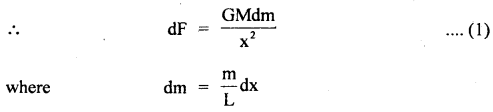
Class 11 Physics Important Questions Chapter 8 Gravitation 90
If F be the total force on the rod, then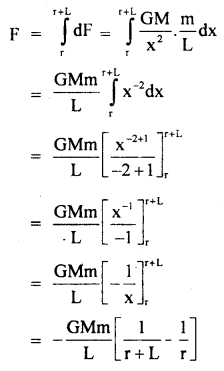
Class 11 Physics Important Questions Chapter 8 Gravitation 91
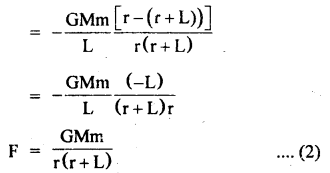
Class 11 Physics Important Questions Chapter 8 Gravitation 92
Now if the rod is very small as compared to its distance from the earth i.e. if r >> L, then r + L ≈ r.

∴ From (2), we get F = GMmr2
i.e. the rod behaves as a point mass lying at a distance r from the center of the earth.

Question 23.
Calculate the scale reading of a spring balance having a body suspended to it and is in a ship sailing along the equator with a speed v.
Answer:
Let m = mass of the body suspended
W0 = scale reading of the spring balance when the ship is at rest

Let ω = angular speed of rotation of the Earth of radius R
∴ The value of g at equator is given by
gθ = (g – Rω2)
∴ W0 = mgθ = m(g – rω2) ….(1)

when the ship sails from west to east with the speed v on Earth’s surface, then its angular speed vR gets added to ω i.e. ω’ = ω + vR
Class 11 Physics Important Questions Chapter 8 Gravitation 93
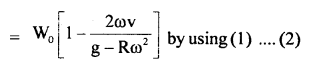
Class 11 Physics Important Questions Chapter 8 Gravitation 94
similarly if the ship sails from east to west, then the scale reading is given by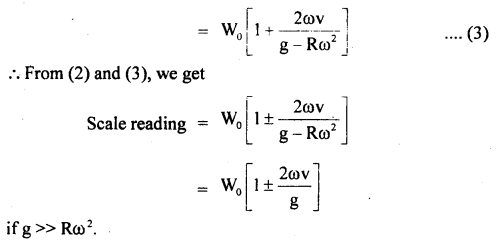
Class 11 Physics Important Questions Chapter 8 Gravitation 95

Question 24.
Calculate the minimum work done in bringing a spaceship of mass 1000 kg from the surface of Earth to the moon.
Answer:
Let M1, M1  = masses of earth and moon respectively.
R1, R2 = radius of earth and moon respectively,
m = mass of spaceship.

If U1 and U2 be the gravitational P.E. of the spaceship on Earth and the moon respectively, then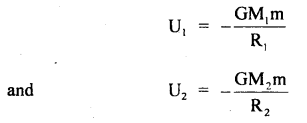
Class 11 Physics Important Questions Chapter 8 Gravitation 96

The total work (W) will have to be done on the spaceship first against the gravity of Earth and-then against the gravity of the moon through the zero-gravity region while going from the Earth to theme on.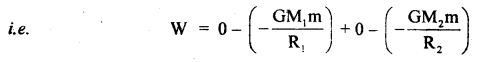
Class 11 Physics Important Questions Chapter 8 Gravitation 97
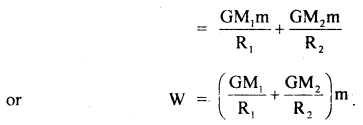
Class 11 Physics Important Questions Chapter 8 Gravitation 98

Question 25.
Taking moon’s period of revolution about the Earth as 30 days, calculate its distance from the Earth;
G = 6.67 × 10-11 Nm2 Kg-2, M = mass of Earth = 6 × 1024 kg.
Answer:
Here, T = 30 days = 30 × 24 × 3600s
= 30 × 86400 s
G = 6.67 × 1011 Nm2Kg-2
M = 6 × 1024 kg

Let m = mass of moon.
Let x = distance of moon from Earth = ?

Here, the force of attraction between moon and Earth provides the centripetal force to the moon i.e.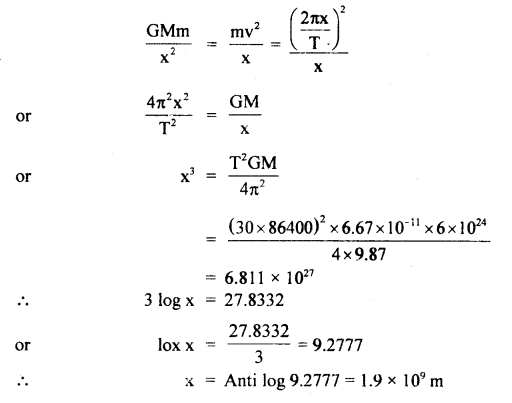
Class 11 Physics Important Questions Chapter 8 Gravitation 99

Value-Based Type:

Question 1.
Suresh was struggling to understand Kepler’s second law of planetary motion. Then his friend Raman who came to him explained how the planet moves around the sun obeying Kepler’s law of planetary motion.
(a) Comment upon the values of Raman.
Answer:
Raman shares his friends and wants to improve his knowledge in the subjects, has a concern towards his friends.

(b) State Kepler’s laws of planetary motion.
Answer:
Kepler’s 1st law of planetary motion: Every planet revolves around Sun in an elliptical orbit with Sun at one of its foci. BacondlawsThe radius vector joining the center of Sun and planet sweeps out equal areas in equal intervals of time i.e. areal velocity of the planet around the Sun always remains constant. Third law: The square of the time period T of revolution of a planet around the Sun is proportional to the cube of the semi-major axis R of its elliptical orbit i.e.
T2 ∝ R3

Question 2.
Suresh went to a picnic on a hill station. The teacher told all the students to weigh by weighing machine before going to the hill station. His weight was 56 Kg. When we reached the hill station along with his friends, our teacher again told us to find their weight using a weighing machine installed in front of a shopping mall. He found that his weight was lesser than 56 Kg i.e 52 Kg, He was surprised and asked the teacher the reason behind it. The teacher explained that the acceleration due to gravity decreases as we go up from the ground. Hence, your weight will be lesser than on the ground.
(i) What values of Suresh are displayed here?
Answer:
Awareness, intelligence, and logic.

(ii) Why does the teacher tell everyone to find their weight?
Answer:
The teacher wanted to explain the acceleration due to gravity through the demonstration method.

(iii) If a body is taken to a height equal to the radius of the earth from its surface, how will the weight of a body change?
Answer:
We know that: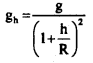
Class 11 Physics Important Questions Chapter 8 Gravitation 100
Class 11 Physics Important Questions Chapter 8 Gravitation 101
Hence, the weight of the body will reduce to one-fourth of its original weight on the surface of the earth.

Question 3.
Two friends Jagat and Ram are discussing escape velocity, Jagat told that if a body is projected vertically upwards with escape velocity, it will cross the earth’s gravitational field and will never come back to the earth’s surface again. Ram was not convinced with Jagat. So, they went to ask their physics teacher about escape velocity. The teacher explained to him about escape velocity:
(i) What values are displayed by Jagat and Ram? Is Jagat’s definition was correct?
Answer:
Yes, Jagat’s definition was correct.
Values are: Curious to know, intelligent, co-operative, helping and sharing their ideas.

(ii) What is the value of escape velocity?
Answer:
We know that:
Ve= 2 gRE−−−−−√
[ On substituting the values g and RE]
= 112 km / s
This is the value of escape speed, sometimes loosely called the escape velocity.

Question 4.
Abhinav and Ankit are in the same section of class 11. Ankit fell sick for two days. So, he could not attend the classes. In two days his physics teacher explained about “Earth Satellites” and “Weightlessness”. Abhinav explained these two topics to Ankit later on Sunday.
(i) Which values are displayed by Abhinav?
Answer:
The values displayed by Abhinav are:
Helping nature, Ability, and Willingness to explain, patience

(ii) Find an expression for the weight of a body at the center of the Earth.
Answer:
We know that the value of acceleration due to gravity at a depth “d” below the surface of the earth is given by at the center of the earth
Class 11 Physics Important Questions Chapter 8 Gravitation 102
∴ Weight of a body at the center of the earth mg’ = m × o = o
So, the body will be weightless.

Label :
Amresh Academy

0 comments:

Subscribe to: Post Comments (Atom)